Factor Analysis evaluates the latent variable. But we do not have the data of latent variables. Other Multi-Variable Analysis methods do not deal with such variable.
Many methods are used to predict or categorize. But Factor analysis is used to evaluate hypothesis.
Latent variables are imaginary variable.
Factor analysis is developed in the field of psychology. The field needs to analyze factors. Latent variables are used as factors in the field.
But today, latent variables are not only for factors.
In this page, I call "explicit variable" as the real variable.
In the eauation, latent variables are the linear function of explicit variables.
Classical Factor Analysis is similar to Principal Component Analysis.
Principal Component Analysis calculate principal component using some rules.
Classical Factor Analysis changes the rule to evaluate the hypothesis of the analyst. If the hypothesis is not good, the calculation does not go well.
Principal Component Analysis is used to get hint to make the hypothesis.
Recent Factor Analysis remove the similarity of Principal Component Analysis.
One of the applications of this analysis is SEM and Covariance Structure Analysis. And the tool of the application can be used for recent factor analysis
An example of R has a page for Factor analysis by R.
In addition to the analysis of the relationship between variables and factors, the analysis of the relationship between samples and factors is also introduced.
For R-EDA1, factor analysis can be performed by selecting "Similarity_of_Variables_and_Categories"-> "Among_all_columns"-> "Factor_Analysis". Enter the number of factors and select the type of rotation.
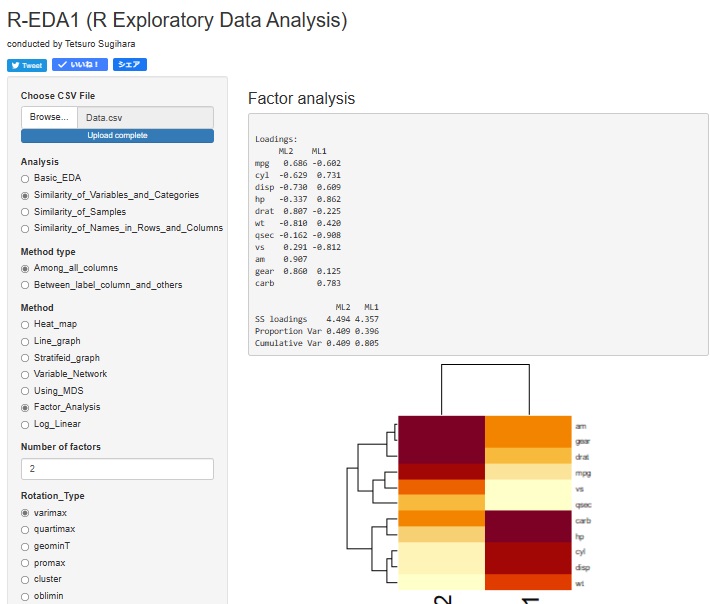
Unlike Factor analysis by R, it seems that heatmaply cannot be used here, so analysis of the relationship between samples and factors is not included at this time.
NEXT 
SEM and Covariance Structure Analysis
