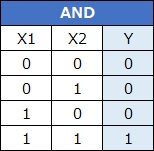
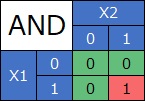
The AND condition is a property that becomes 1 only when the two are combined.
Combinations such as "salt in soup powder" and "salt in watermelon" are said to "increase the sweetness" despite the addition of salt. In this way, what does not happen in one thing happens in combination is called "interaction" in data science. Synergy or countervailing is a type of interaction.
Phenomena with interactions complicate the relationship between cause and effect. The difficulty of causal inference is high, so it is difficult to improve and manage. Therefore, for example, in Quality Engineering, we use Mixed Orthogonal Array to try to find the effects that can be seen by individual variables without dealing with phenomena with strong interactions.
On the other hand, one field that has been studying the effects of interactions since ancient times is Oriental Medicine. Over the years, and with the involvement of many people, we have accumulated knowledge of interactions that are useful in medicine. We analyze how synergies and countervailing effects occur, and some, such as herbal medicines, take advantage of synergies that occur in complex combinations.
Before we talk about interactions, let's talk about synergies that are not interactions.
For example, "Person A can make 10 pieces a day, and Person B can make 10 pieces a day. If you make it with just one person, you can only make 10 pieces a day, but if you make it with two people, you can make 20 pieces a day." In this way, an effect that can be expressed by addition may be a kind of synergy in the everyday sense, but it is not an interaction.
In this example, if there is an interaction, it is "If two people make it, you can make 40 pieces a day", "Even if two people make it, you can only make 10 pieces a day", or "If you make it with two people, you can only make 5 pieces a day". If there are more than 20, there is a synergistic interaction, and if there are fewer than 20, there is a countervailing interaction.
There are different types of interactions. As a first classification, logical calculations seem to be good.
The 0 and 1 in the figure below are used by reading, for example, "0 is no countermeasure, 1 is countermeasure".


The AND condition is a property that becomes 1 only when the two are combined.
This is an effect known as "synergy." If there is only one countermeasure, nothing will happen, but if two or more measures are met, the effect will occur only if it is met.
An example of this is herbal medicine. No matter how much you research individual herbal medicines, you will not know the effect, but the combination of multiple herbal medicines will produce the effect as a medicine.
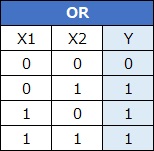
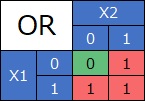
The OR condition is a property that if it is one of the two, it will be 1.
It is similar to the phenomenon that can be expressed by the introductory multiple regression equation, except that it is "1" even if both are 1. The introductory multiple regression analysis equation is different from the OR condition because it can handle a phenomenon that becomes "1" if there is only one, and "2" if two are combined.
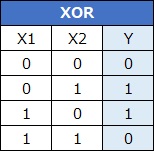
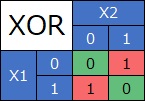
The XOR condition is the same as that of OR, but it is a property that becomes "0" even if it is one of the two.
The phenomenon of "offsetting" applies. When you use the Design of Experiments, there is a story that "interactions appear in this column of the orthogonal table, so be careful how you use them." The interaction at the time of that talk is that of the XOR condition.
NEXT 

 Force of Interaction term
Force of Interaction term
